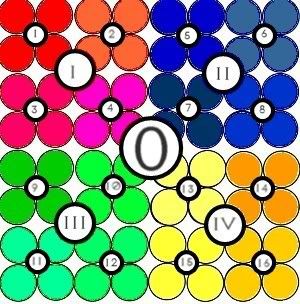
Pity about the search function being down; I know there was a topic on this a while back but I can't find it. Sorry if this idea has been done before. Anyway, the question was "how are the 64 cogs of Regulus arranged?" We know that one of them is in the center, so the other 63 cogs must have some kind of symmetry about that one. It's also a requirement that the cogs be physically able to rotate against each other (no locked groups of odd numbers of gears in loops). I thought about this for a bit and came up with:
http://earl.of.sandwich.net/Mechanus.pdf .
(My art skills will not be showing up in Artist's Alley any time soon. ;^) )
Each circle represents a gear; regions (4 gears), and quarters (16 gears) are also color-coded, with regions being hue variants on the main color of the quarter and the central gear being singled out.
This is one of the most geometrically degenerate possible arrangements, actually -- while its simplicity might appeal to the modron mind, I'm trying to come up with something more complex and hopefully aesthetically pleasing. Three dimensions would rock; I'm fiddling around with gears on the faces of octahedrons and cubes but it's slower going.



Your configuration fits the basic requirements pretty well.
I'll note that Planes of Law describes Regulus this way:
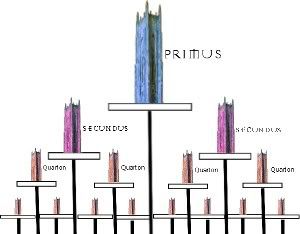
"The 64 cogs of Regulus seem to be stacked in a pyramid, if viewed from the side. A huge rod, nearly as thick around as the spire in the Outlands, runs through the center of these gears and is apparently the agent by which they turn (if they don't turn it instead)."
The earlier thread (with my take) is here. Strictly speaking, my version has more than 64 gears, though the lowest tier encompasses precisely 64.